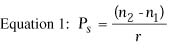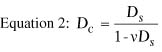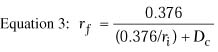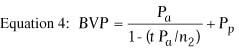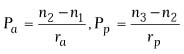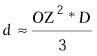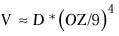| Because most lamellar refractive procedures alter the anterior corneal
surface, accurate information about this surface is essential in planning
and analyzing these procedures. Early on it was found that changes
in keratometry correlated poorly with the change in refraction observed.1,2,3 One hypothesis concerns the fact that the keratometer fits a curve through
two points, approximately 3 mm apart, in each of two axes, assuming
that the cornea is perfectly spherocylindrical between the points. This
reading often does not reflect the postoperative corneal surface
power accurately over the visual axis, where irregularity and multifocality
may be present. It is also important to recall that the dioptric power given by the keratometer
is an estimate of the total corneal power based on the measured
radius of curvature and assuming normal corneal thickness and posterior
corneal curvature. Therefore, if the keratometer is used, the anterior
surface curvature measurements are more reflective of the refractive
change. Keratoscopy provides more information about the shape and refractive power
of the corneal surface than does keratometry (Fig. 1).4 Significantly more detail can be obtained by computer analysis of such
images. Most currently used systems for corneal topographic examination
utilize Placido disc images. Multiple illuminated concentric rings
are placed in front of the cornea, and the reflections of the rings are
viewed and analyzed. Corneal curvature can be determined at many points
along each ring, giving a detailed image of the corneal surface power (Fig. 2). Unfortunately, this also requires some assumptions about corneal
shape and may be less accurate on aspheric surfaces. Elevation maps
can also be derived from the curvature data. 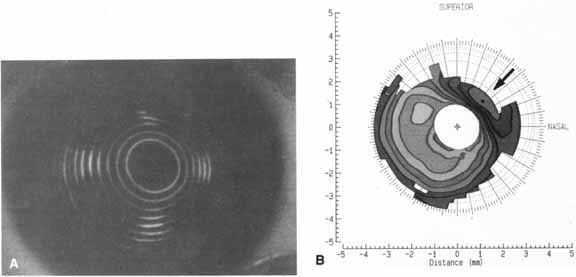 Fig. 1 A. Keratoscope photograph of a cornea 1 year after epikeratophakia for myopia. With
a refraction of -1.50 + 1.00 × 50°, the
visual acuity is 20/25 +. B. Keratometry reveals 6 D of astigmatism at 45°, which is evident in
the keratoscope photograph (arrow). (Courtesy of Stephen D. Klyce, Ph.D., and Steven A. Dingeldein, M.D., LSU
Eye Center, New Orleans) Fig. 1 A. Keratoscope photograph of a cornea 1 year after epikeratophakia for myopia. With
a refraction of -1.50 + 1.00 × 50°, the
visual acuity is 20/25 +. B. Keratometry reveals 6 D of astigmatism at 45°, which is evident in
the keratoscope photograph (arrow). (Courtesy of Stephen D. Klyce, Ph.D., and Steven A. Dingeldein, M.D., LSU
Eye Center, New Orleans)
|
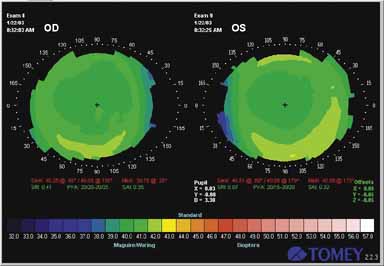 Fig. 2 Computerized analysis of corneal topography after LASIK for myopia. Note
central flattening in each eye. Fig. 2 Computerized analysis of corneal topography after LASIK for myopia. Note
central flattening in each eye.
|
Many other non-Placido disc systems have been used for corneal topography
analysis. The most commonly used today (Orbscan, Bausch & Lomb, Rochester, NY) is based on analysis of multiple Scheimpflug slit images of the cornea. Anterior
corneal elevation, corneal thickness, and posterior corneal
shape can be determined. LAMELLAR REFRACTIVE KERATOPLASTY PROCEDURES Refractive keratoplasty can be divided into lamellar procedures and nonlamellar
procedures. Nonlamellar procedures alter corneal curvature without
the addition or removal of tissue. Radial keratotomy and various
types of incisional surgery for astigmatism are the most common nonlamellar
procedures. Lamellar procedures involve the removal of tissue from the cornea or the
addition of tissue or synthetic material to change its refractive power. Most
lamellar procedures change the refractive power by altering
the anterior corneal curvature, which can be accomplished in several ways. A
tissue or hydrogel lens can be placed within the corneal stroma (keratophakia
and intrastromal hydrogel lens implantation); a
tissue lens can be placed on the anterior corneal surface (epikeratophakia); the
anterior lamellae of the cornea can be removed, shaped, and
replaced (keratomileusis); or the excimer laser
can be used to remove corneal stroma, either on the surface or within
the stroma. In one lamellar procedure (intrastromal polysulfone lens implantation), a synthetic lens
with a high refractive index was placed within the cornea and changed
the refractive properties of the cornea without altering the anterior
corneal curvature. Each of these procedures is briefly described in the following sections. KERATOPHAKIA AND INTRASTROMAL LENS IMPLANTATION Modern lamellar refractive keratoplasty originated with Jose I. Barraquer's
work in Colombia, South America. In 1949, Barraquer developed
keratophakia for the correction of aphakia because of the dissatisfaction
with aphakic spectacles and the contact lenses and intraocular lenses
that were available at that time. His results were first reported
in 1963,5 and the procedure was introduced in the United States in 1977.6 Attempts to adapt Barraquer's classic form of keratophakia for the
correction of myopia have not been successful. In keratophakia, a plus-powered tissue lens of donor corneal stroma
is implanted intrastromally into the patient's cornea (Fig. 3). The portion of the patient's cornea that overlies the implanted
tissue lens conforms to the anterior surface of the tissue lens, and
the anterior corneal curvature steepens. 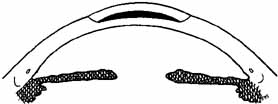 Fig. 3 Keratophakia. An anterior section of the patient's cornea is removed
and replaced over a tissue lens of donor corneal stroma. The anterior
corneal surface is steepened. Fig. 3 Keratophakia. An anterior section of the patient's cornea is removed
and replaced over a tissue lens of donor corneal stroma. The anterior
corneal surface is steepened.
|
Prior to surgery, the donor corneal stroma is lathed with a cryolathe, which
is a modified contact lens lathe that keeps the tissue frozen as
it is being lathed. At the time of surgery, an anterior lamellar section
of tissue is removed from the patient's cornea with a microkeratome, which
is similar to a carpenter's plane. The prelathed tissue
lens is placed on the exposed stromal bed, and the anterior lamellar
section that was previously removed from the patient's cornea
is sutured back into place on top of the tissue lens. It is necessary
to remove an anterior lamellar section of tissue from the patient's
cornea before implanting the tissue lens. If the tissue lens is placed
in a lamellar pocket without a 360° anterior incision that penetrates
Bowman's layer, there is little effect on the anterior corneal
curvature;7,8 unless Bowman's layer is severed completely, the posterior layers
of the cornea change shape rather than the relatively inelastic anterior
layers (Fig. 4). 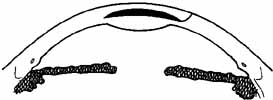 Fig. 4 If an anterior section of the patient's cornea is not removed and
the tissue lens is implanted in a lamellar pocket, the posterior rather
than the anterior corneal surface is altered. Fig. 4 If an anterior section of the patient's cornea is not removed and
the tissue lens is implanted in a lamellar pocket, the posterior rather
than the anterior corneal surface is altered.
|
Changes in anterior corneal curvature and refractive power have also been
achieved with intrastromal hydrogel lenses, which have a refractive
index (1.381) similar to that of the cornea. Both plus-powered9–11 and minus-powered12 hydrogel lenses have survived implantation into the corneas of experimental
animals by means of the keratophakia technique, but the accuracy
of the optical results and the long-term safety were not sufficient
to warrant clinical use. Intrastromal polysulfone lenses have also been used to correct hyperopia
and myopia.13,14 Polysulfone has a high refractive index (1.633); a plus or minus
lens can be made from this material and implanted in a pocket in
the corneal stroma. Unlike tissue lenses and hydrogel lenses, which change
the refraction of the cornea by altering the curvature of the anterior
corneal surface, polysulfone lenses change the refractive power
of the cornea by altering the path of light as it passes through the cornea–lens
interface within the cornea. No change in the anterior
corneal curvature is necessary to achieve this effect. Therefore, these
lenses can be implanted into a lamellar pocket without the need for
removing an anterior lamellar section of tissue. KERATOMILEUSIS In 1964, Barraquer developed keratomileusis for the correction of myopia.15 He later adapted the technique for the correction of hyperopia and first
reported his results in 1981.16 In keratomileusis, an anterior lamellar section of tissue is removed from
the patient's cornea with a microkeratome (Fig. 5). This tissue is lathed, from the posterior surface, on a cryolathe
and is then sutured back onto the stromal bed (Fig. 5). If more tissue is removed from the periphery of the tissue than from the
center, the anterior corneal curvature steepens; if more tissue is removed
from the center than from the periphery, the anterior corneal curvature flattens. This autoplastic keratomileusis
procedure requires no donor tissue. However, a prelathed donor tissue
lens can be used, in which case the procedure is called homoplastic keratomileusis. 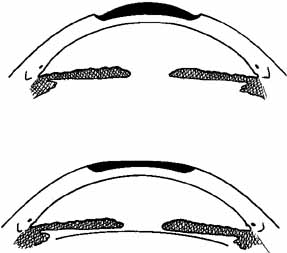 Fig. 5 Keratomileusis for aphakia (top) and myopia (bottom). An anterior section of the patient's cornea is removed, shaped from
the posterior surface, and replaced, altering the anterior corneal
surface. Fig. 5 Keratomileusis for aphakia (top) and myopia (bottom). An anterior section of the patient's cornea is removed, shaped from
the posterior surface, and replaced, altering the anterior corneal
surface.
|
EPIKERATOPHAKIA Epikeratophakia evolved from the Barraquer procedures of keratophakia and
keratomileusis. It was developed by Kaufman and co-workers17 to provide a procedure that was safer and simpler than keratophakia or
keratomileusis. The results of epikeratophakia for aphakia were first
published in 198118 and those of epikeratophakia for myopia in 1985.19 Epikeratophakia was used widely for a number of years, but was largely
abandoned with the development of superior techniques.20–22 In epikeratophakia, a prelathed tissue lens of donor corneal stroma and
Bowman's layer is sutured onto the anterior surface of the patient's
cornea (Fig. 6). The tissue lens was lathed preoperatively at a central laboratory, according
to the patient's preoperative measurements (refraction
at the corneal plane and average keratometry). Plus-powered
and minus-powered tissue lenses were available. Both
had a central optical zone and a peripheral wing. After being lathed, the
tissue was lyophilized and shipped to the surgeon. At the time of
surgery, it was rehydrated. 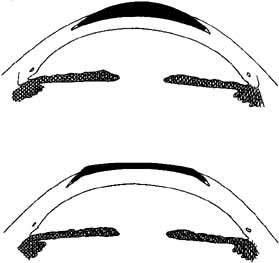 Fig. 6 Epikeratophakia for aphakia (top) and myopia (bottom). A tissue lens of donor corneal stroma and Bowman's layer is placed
on the patient's cornea, which has been deepithelialized centrally. The
wing of the tissue lens is placed into a peripheral lamellar
pocket. Fig. 6 Epikeratophakia for aphakia (top) and myopia (bottom). A tissue lens of donor corneal stroma and Bowman's layer is placed
on the patient's cornea, which has been deepithelialized centrally. The
wing of the tissue lens is placed into a peripheral lamellar
pocket.
|
The central epithelium of the patient's cornea is removed, and a peripheral
lamellar keratotomy is created into which the wing of the tissue
lens is inserted and then sutured. The anterior surface of the tissue
lens forms the anterior surface of the patient's cornea. The
tissue lens is held in place by a thin peripheral scar. Because the central
Bowman's layer of the patient's cornea is undisturbed, a
central scar does not form, which allows the tissue lens to be removed
and the procedure to be repeated if desired. Tissue Lathing In keratomileusis and epikeratophakia, the tissue lens forms the anterior
surface of the eye postoperatively. This surface must retain its ability
to support an epithelial layer and smooth tear film. For this reason, attempts
are made to retain the anterior structures of the tissue
lens, including the epithelial basement membrane and Bowman's layer. Therefore, lathing
is done on the posterior surface. This altered
posterior surface conforms to the unaltered corneal bed, and the anterior
surface of the tissue lens takes on a new configuration (Fig. 7). 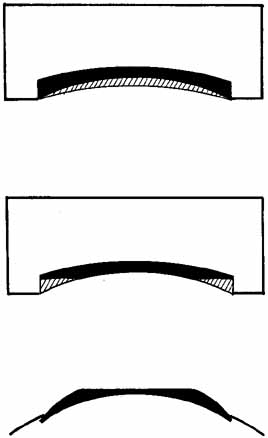 Fig. 7 (Top) A tissue lens can be shaped by placing the anterior surface of the cornea
on a plastic base and removing tissue from the posterior surface (hatched area). More tissue is removed centrally to create a lens for myopia. (Center) Additional tissue is removed from the periphery to create a wing. (Bottom) When placed on the patient's cornea, the posterior surface of the
tissue lens conforms to the anterior surface of the patient's cornea, and
the anterior surface of the tissue lens assumes a different
shape. In this method of shaping a tissue lens, the plastic base remains
constant, and the lathing parameters for the posterior surface are
varied. Fig. 7 (Top) A tissue lens can be shaped by placing the anterior surface of the cornea
on a plastic base and removing tissue from the posterior surface (hatched area). More tissue is removed centrally to create a lens for myopia. (Center) Additional tissue is removed from the periphery to create a wing. (Bottom) When placed on the patient's cornea, the posterior surface of the
tissue lens conforms to the anterior surface of the patient's cornea, and
the anterior surface of the tissue lens assumes a different
shape. In this method of shaping a tissue lens, the plastic base remains
constant, and the lathing parameters for the posterior surface are
varied.
|
More detailed descriptions of a method for calculating lathing parameters
have been published.23–25 All these calculations assume that the tissue lens is placed in its bed
without tension or distortion, that there is no change in its refractive
index, and that the cornea posterior to the tissue lens is not altered
by the procedure. It is difficult to suture the tissue lens without
tension or distortion, and irregular astigmatism as well as undercorrection
or overcorrection may result. The validity of the other assumptions
has not been tested. The freezing process causes dimensional alterations in the lathing apparatus
as well as in the corneal tissue. Both the rotating headstock and
cutting tool contract, and the tissue decreases in diameter and increases
in thickness. Because accuracy to at least 0.1 mm is required, these
alterations must be taken into account. Freezing also damages the
tissue, particularly the keratocytes.25–26 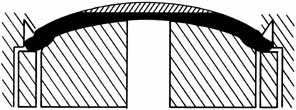 Fig. 8 A tissue lens can be shaped by varying the plastic base and keeping the
method of removal of posterior tissue constant. Fig. 8 A tissue lens can be shaped by varying the plastic base and keeping the
method of removal of posterior tissue constant.
|
PHOTOREFRACTIVE KERATECTOMY (PRK) In the late 1980s researchers began to shape the corneal surface using
an excimer laser. This laser emits light with a wavelength of 193 nm, which
ablates surface tissue without injury to adjacent tissue.27 The laser emits a 6.5 mm pulsed beam that removes approximately 0.25 microns
of tissue. Removal of more tissue centrally than peripherally can
flatten the central corneal curvature and correct myopia (Fig. 9). Initially this was accomplished by a computer-controlled
diaphragm that opened as the procedure progressed, such that the peripheral
treated area was exposed for the least time and the central cornea
for the greatest time. Astigmatism could be corrected by removal of
tissue in one meridian using a rectangular beam. Since then scanning
spot lasers have also been developed. These can also be used to treat
hyperopia by removal of tissue in the corneal midperiphery (Fig. 10). 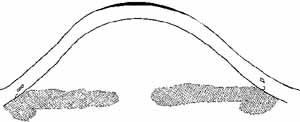 Fig. 9 Diagram of photorefractive keratectomy for myopia. Dark area indicates
tissue removed with the excimer laser in order to flatten central curvature. Fig. 9 Diagram of photorefractive keratectomy for myopia. Dark area indicates
tissue removed with the excimer laser in order to flatten central curvature.
|
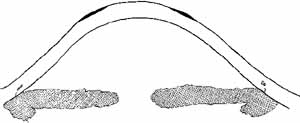 Fig. 10 Diagram of photorefractive keratectomy for hyperopia. Dark area indicates
tissue removed with the excimer laser in order to steepen central curvature. Fig. 10 Diagram of photorefractive keratectomy for hyperopia. Dark area indicates
tissue removed with the excimer laser in order to steepen central curvature.
|
In the mid-1990s researchers began to apply the ablation under a
lamellar stromal flap created with a microkeratome (Fig. 11).28 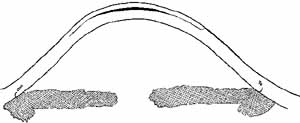 Fig. 11 Diagram of laser-assisted in situ keratomileusis (LASIK). A
large central lamellar flap is created and lifted. Dark area indicates
tissue removed with the excimer laser in order to flatten central
curvature for myopia. Fig. 11 Diagram of laser-assisted in situ keratomileusis (LASIK). A
large central lamellar flap is created and lifted. Dark area indicates
tissue removed with the excimer laser in order to flatten central
curvature for myopia.
|
OPTICAL ISSUES Centration Several optical problems must be addressed to optimize the refractive results
of these procedures. First, how should the optical correction be
centered? There is no simple way to identify the corneal intercept
of the visual axis. The current consensus is that procedures be centered
on the entrance pupil, the virtual image of the real pupil formed
by the cornea.29 The entrance pupil is approximately 0.5 mm anterior to and 14% larger
than the real pupil.30 Unfortunately, the center of the entrance pupil can vary with changes
in the diameter of the pupil. Others have recommended using the location
of the corneal reflex of a fixation light, when viewed by both of the
surgeon's eyes. Determination of Desired Correction
For any of the lamellar refractive keratoplasty procedures, the desired
amount of power correction at the corneal plane must be determined. In
most cases, the desired postoperative result is emmetropia. However, in
some cases ametropia may be preferable, depending on the refractive error
of the fellow eye. The correction at the corneal plane can be calculated
from the spectacle correction, if the vertex distance is known, by means
of the following equation:
where Dc is the correction at the corneal plane in diopters, Ds is the correction at the spectacle plane in diopters, and v is the vertex distance in meters. Determination of Desired Postoperative Anterior Corneal Curvature
The desired postoperative radius of anterior corneal curvature can be
calculated with the following equation, which is derived from Equation
1:
where rf is the postoperative radius of anterior
curvature in meters, ri is the preoperative radius
of anterior curvature in meters, and Dc is the
correction at the corneal plane in diopters.
Equation 3 is for a thin lens and does not take into account
the change in corneal thickness that occurs with lamellar refractive keratoplasty.
In epikeratophakia and keratophakia, the central corneal thickness is
increased; in PRK, LASIK, and keratomileusis, it is decreased. In epikeratophakia
for aphakia, for example, the central corneal thickness increases from
0.52 mm to approximately 0.86 mm.1 The back
vertex power of a thick lens, such as the cornea, is given by the following
equation:
where
and where BVP is the back vertex power of the cornea in diopters,
Pa is the power of the anterior surface in diopters,
Ppis the power of the posterior surface in diopters,
ra is the radius of anterior curvature in meters,
rp is the radius of posterior curvature in meters,
and t is the thickness of the cornea in meters. In this equation,
n1 is the refractive index of air (1.000), n2
is the refractive index of the cornea (1.376), and n3
is the refractive index of aqueous humor (1.336).
In a hypothetical epikeratophakia patient with a preoperative radius of
anterior curvature of 7.7 mm and a desired correction of +15.00 D, Equation 3 predicts that the desired postoperative radius of anterior curvature would
be 5.9 mm. However, with this new anterior curvature and increased
corneal thickness, the back vertex power of the cornea would increase
by 16.6 D instead of by the desired 15.0 D. It is evident that for the
relatively high refractive changes achieved with lamellar refractive
keratoplasty, alterations in corneal thickness should be taken into
account. Barraquer, in his keratomileusis calculations, used an estimated
effect of the change in corneal thickness: the final radius of anterior
curvature calculated by Equation 3 is reduced by 0.004095 mm for each diopter of myopic correction. Finally, as the position of the anterior surface of the eye is moved, the
effective axial length of the eye is also altered. This effect is taken
into account if back vertex power of the cornea is used. Determining Amount of Tissue Removal
The following formula can be used to calculate how much of the axial
corneal stroma must be removed to achieve the desired myopic correction:
 |
 |
| where d = the maximum
depth of ablation, R1 = the initial radius of curvature
in millimeters, D = the diopters of correction, and OZ
is the diameter of the optical zone of the correction in millimeters.
|
Munnerlyn indicated that the ablation depth can be approximated using 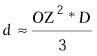
It is evident from this formula that the depth of the ablation is directly
proportional to the diopters of correction and proportional to the square
of the optical zone (Fig. 12).
For a 6 mm optical zone, a 3 diopter correction needs to ablate approximately
36 microns of tissue, and a 9 diopter correction needs to remove approximately
108 microns. To achieve a 9 diopter correction with an optical zone of
5 mm, the depth of ablation is approximately 75 microns; increasing the
optical zone to 8 mm increases the depth to 192 microns.
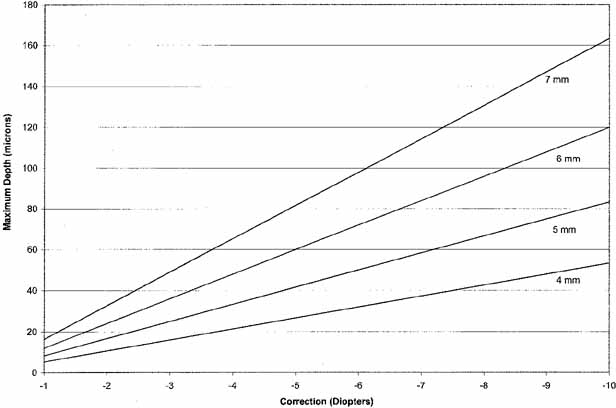 Fig. 12 Relationship between diopters of correction for myopia, optical zone diameter, and
maximum depth of ablation, in microns. Fig. 12 Relationship between diopters of correction for myopia, optical zone diameter, and
maximum depth of ablation, in microns.
|
The volume of tissue removed can be estimated by the formula31
where V = the volume of tissue removed.
Minimum Optical Zone Theoretically the larger the optical zone the better. However, some physical
constraints limit the diameter of the optical zone that can be achieved. As
can be seen from the previous section, the depth of tissue
removal increases dramatically as the optical zone is increased. Tissue
removal is constrained by two factors: If you remove too much tissue
the cornea is weakened and can become ectatic; in phototherapeutic keratectomy
the greater the depth of ablation the greater the risk of postoperative
scarring, and in LASIK the greater the depth the greater
the risk of irregular astigmatism. The risk of postoperative glare and starburst is primarily related to the
spherical equivalent correction, but is also related to the relative
size of the optical zone and pupil. The smaller the optical zone is
in relation to the pupil size, the greater the experience of these symptoms.32–34 Therefore, optimally the optical zone should be at least the size of the
entrance pupil in dim illumination.35 In my experience the optical zone can be 0.5 mm smaller than the pupil
without a high risk of symptomatic glare. The risk of scarring and glare also appears to be related to the shape
of the transition zone between the optical treatment and the peripheral
cornea. A more gradual transition decreases the likelihood of both these
complications.36 This can be achieved by creating an aspheric ablation, with a transition
zone extending 1–2 mm beyond the optical zone. The VISX (address) software creates a “blend” zone by treating 1 diopter of the
myopic correction with an 8.0 mm optical zone and the remainder with
a 6.0 or 6.5 mm optical zone. The blend zone only increases the central
depth of the ablation by 9 microns. This appears to significantly
reduce the incidence of glare in patients with scotopic pupils of 7 to 8 mm. Limitations of Lathing Procedures There are physical limitations to the amount of correction that can be
obtained by each of the lamellar refractive keratoplasty procedures. The
thickness of the tissue lens is limited by the thickness of the corneal
tissue from which it is made. If a donor cornea is used, the full
stromal thickness (approximately 0.49 mm) is available. However, the
maximum thickness of an anterior lamellar section from a patient's
cornea is approximately 0.4 mm. With a minimum optical zone
diameter of 5 mm, the amount of aphakic correction that can be obtained
with keratomileusis is limited to approximately 12 D to 15 D when
a lamellar section is used (autoplastic keratomileusis). Depending
on the preoperative corneal curvature, an additional 4 D to 6 D
could be obtained when a full-thickness donor cornea is used (homoplastic
keratomileusis). The amount of myopic correction
that can be obtained is approximately 15 D with autoplastic keratomileusis
and 22 D with homoplastic keratomileusis. The maximum amount of
aphakic correction with keratophakia is approximately 20 D. The maximum
corrections that have been achieved with epikeratophakia for aphakia
and myopia are 34 D and 38 D, respectively. Limitations on Postoperative Corneal Curvature Barraquer has stated, based on his experience, that there are limits on
the postoperative thickness and anterior curvature of the cornea, as
well as a minimum thickness of the lathed tissue lens. He noted that, after
keratophakia, the maximum corneal thickness that is consistent with
good vision is 0.70 mm. The maximum anterior corneal curvature after
keratophakia or keratomileusis for aphakia is 58 D. The minimum anterior
corneal curvature after keratomileusis for myopia is 33 D. The minimum
central thickness of the tissue lens is 0.12 mm in keratophakia
and 0.09 mm in keratomileusis. In epikeratophakia the minimum central
thickness of the tissue lens is approximately 0.1 mm. Although some have suggested that excessively steep or flat postoperative
corneal curvatures after PRK or LASIK are associated with decreased
vision, a recent study did not observe this.37 There appears to be no maximum or minimum postoperative corneal curvature
or maximum corneal thickness that is consistent with good vision. |